OFFICE LINE
1.800.555.6789
EMERGENCY
1.800.555.0000
WORKING HOURS
9:00am – 6:00pm
OFFICE LINE
1.800.555.6789
EMERGENCY
1.800.555.0000
WORKING HOURS
9:00am – 6:00pm
ABOUT US
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Vestibulum vestibulum quis metus sodales ullamcorper. Vivamus eu sodales sapien, sit amet rhoncus nisi. Curabitur luctus ipsum ac magna ultricies imperdiet.
ELECTRICAL SERVICES
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Vestibulum vestibulum quis metus sodales ullamcorper. Vivamus eu sodales sapien, sit amet rhoncus nisi. Curabitur luctus ipsum ac magna ultricies imperdiet.
TESTIMONIALS
You we’re very professional and quick. We will recommend your services to our friends.
Thank you for your very professional and promt response. I wish I had found you before I spent money on a competitors theme.
We had floods in our town and we lost our electricity. You and your team got us back up and running in no time. Thanks Avada!
OUR BLOG
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Vestibulum vestibulum quis metus sodales ullamcorper. Vivamus eu sodales sapien, sit amet rhoncus nisi. Curabitur luctus ipsum ac magna ultricies imperdiet.
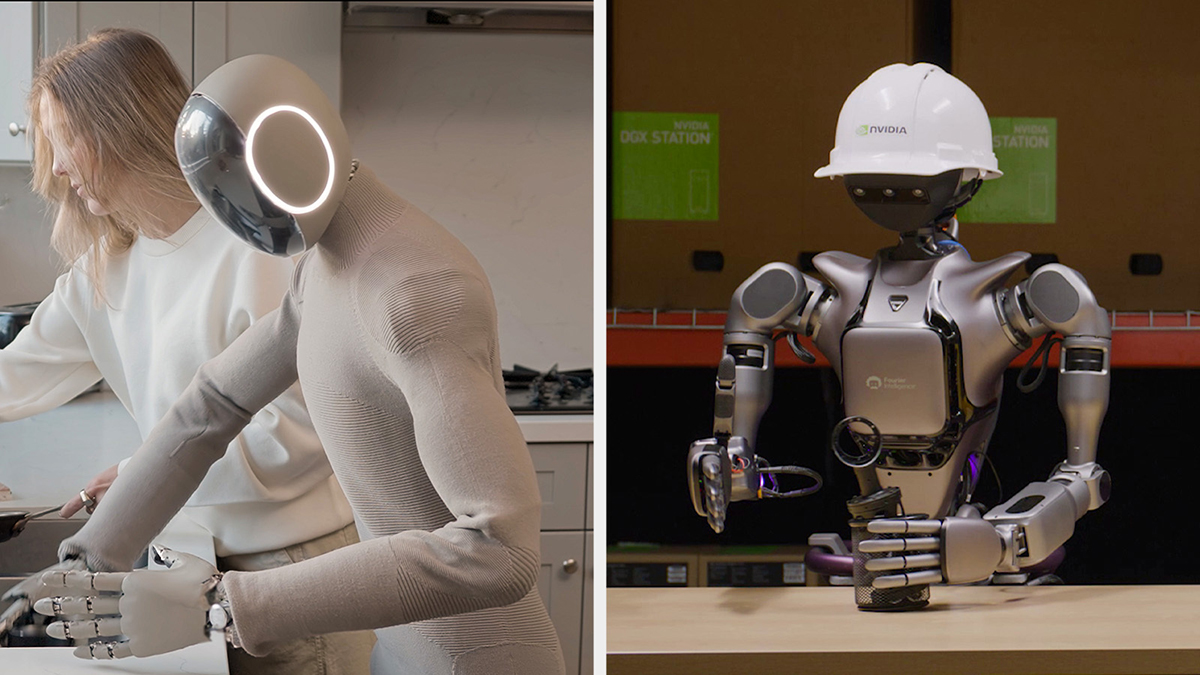
To solve for the given question, we need to find the derivative of the function $f(x) = 3x^2 – 4x + 1$.The derivative of a function $f(x)$ with respect to $x$, denoted as $f'(x)$, represents the rate of change of $f(x)$ with respect to $x$. To find the derivative of $f(x) = 3x^2 – 4x + 1$, we need to apply the power rule for differentiation. The power rule states that if $f(x) = x^n$, where $n$ is a real number, then $f'(x) = nx^{n-1}$.Let us apply this rule term by term for $f(x) = 3x^2 – 4x + 1$.1. For the term $3x^2$: The power rule applied to $x^2$ gives us $2x^{2-1} = 2x$. However, we need to keep the constant coefficient $3$ in place. Thus, the derivative of $3x^2$ is: $$ \frac{d}{dx}(3x^2) = 3 \cdot \frac{d}{dx}(x^2) = 3 \cdot 2x = 6x. $$2. For the term $-4x$: The power rule applied to $x$ (which is $x^1$) gives us $1x^{1-1} = 1 \cdot x^0 = 1$ (since $x^0 = 1$ for $x \neq 0$). However, we need to keep the constant coefficient $-4$ in place. Thus, the derivative of $-4x$ is: $$ \frac{d}{dx}(-4x) = -4 \cdot \frac{d}{dx}(x) = -4 \cdot 1 = -4. $$3. For the constant term $1$: The derivative of any constant term is zero. Thus, the derivative of $1$ is: $$ \frac{d}{dx}(1) = 0. $$4. Combining all the terms together, the derivative $f'(x)$ is: $$ f'(x) = 6x – 4 + 0 = 6x – 4. $$So, the derivative of $f(x) = 3x^2 – 4x + 1$ is: $$ \boxed{6x – 4}. $$To verify, let us recall a few more details about the power rule explicitly for a term $ax^n$ where $a$ is a constant: $$ \frac{d}{dx}(ax^n) = a \cdot nx^{n-1}. $$ This makes it easier to directly apply the rule to each term individually.### Verification by First Principles (if needed for a deeper understanding)The derivative can also be defined as: $$ f'(x) = \lim_{h \to 0} \frac{f(x+h) – f(x)}{h}. $$ For $f(x) = 3x^2 – 4x + 1$: $$ f(x+h) = 3(x+h)^2 – 4(x+h) + 1. $$ Expanding $3(x+h)^2$: $$ 3(x+h)^2 = 3(x^2 + 2xh + h^2) = 3x^2 + 6xh + 3h^2. $$ So, $$ f(x+h) = 3x^2 + 6xh + 3h^2 – 4(x+h) + 1 = 3x^2 + 6xh + 3h^2 – 4x – 4h + 1. $$ We need to find $f(x+h) – f(x)$: $$ f(x+h) – f(x) = (3x^2 + 6xh + 3h^2 – 4x – 4h + 1) – (3x^2 – 4x + 1). $$ $$ = 3x^2 + 6xh + 3h^2 – 4x – 4h + 1 – 3x^2 + 4x – 1. $$ $$ = 3x^2 – 3x^2 + 6xh + 3h^2 – 4x + 4x – 4h + 1 – 1. $$ $$ = 6xh + 3h^2 – 4h. $$ $$ = h(6x + 3h – 4). $$ Now, substitute this back into the limit definition of the derivative: $$ f'(x) = \lim_{h \to 0} \frac{f(x+h) – f(x)}{h} = \lim_{h \to 0} \frac{h(6x + 3h – 4)}{h}. $$ $$ = \lim_{h \to 0} (6x + 3h – 4). $$ $$ = 6x + 3(0) – 4. $$ $$ = 6x – 4. $$ This confirms our result once again.### Conclusion The derivative of $f(x) = 3x^2 – 4x + 1$ is: $$ \boxed{6x – 4}. $$ \boxed{6x – 4}\boxed{“Fix4Bot.com Aims to Repair and Support Nvidia’s ‘Supercharged’ Humanoid Robot Developments”}
\boxed{```html Nvidia has unveiled a foundation model to boost robot reasoning and skills in humanoid robots. How Fix4Bot.com Can Diagnose and Repair Any Dama[...]
The Rise of the Humanoid Workforce: Diagnosing and Repairing the Next Generation of Robots (Apollo, Ameca, Optimus & Beyond) The narrative around humanoid[...]
FREE QUOTE
Do you need help with electrical maintenance? Fill out this form to get in touch.
OFFICE LINE
1.800.555.6789
EMERGENCY
1.800.555.0000
WORKING HOURS
9:00am – 6:00pm
OFFICE LINE
1.800.555.6789
EMERGENCY
1.800.555.0000
WORKING HOURS
